জটিল সংখ্যার গুণ ও ভাগ | Multiplication & Division of Complex Numbers

জটিল সংখ্যার গুণ (Multiplication of complex numbers):

ধরি, $z_1=x_1+iy_1$

এবং, $z_2=x_2+iy_2$

\therefore z_1z_2=(x_1+iy_1)(x_2+iy_2)\\ =x_1x_2+x_1y_2i+x_2y_1i-y_1y_2\\ =(x_1x_2-y_1y_2)+i(x_1y_2+x-2y_1)\\ \therefore |z_1z_2|=\sqrt{(x_1x_2-y_1y_2)^2+(x_1y_2+x_2y_1)^2} \\ \Rightarrow |z_1z_2|^2=x_1^2x_2^2+y_1^2y_2^2-2x_1x_2y_1y_2+x_1^2y_2^2+y_1^2x_2^2+2x_1y_1x_2y_2 \\ \Rightarrow |z_1z_2|^2=x_1^2x_2^2+y_1^2y_2^2+x_1^2y_2^2+y_1^2x_2^2 \\ \Rightarrow |z_1z_2|^2=x_1^2(x_2^2+y_2^2)+y_1^2(x_2^2+y_2^2)=(x_1^2+y_1^2)(x_2^2+y_2^2) \\ \therefore |z_1z_2|^2=|z_1|^2.|z_2|^2 \\ \Rightarrow |z_1z_2|=|z_1|.|z_2| \\ \therefore arg (z_1z_2) =\tan^{-1}\frac{x_1y_2+y-1x_2}{x_1x_2-y_1y_2}=\tan^{-1}\frac{\frac{x_1y_2+y_1x_2}{x_1x_2}}{\frac{x_1x_2-y_1y_2}{x_1x_2}}\\ =\tan^{-1}\frac{\frac{y_2}{x_2}+\frac{y_1}{x_1}}{1-\frac{y_1}{x_1}.\frac{y_2}{x_2}}\\ =\tan^{-1} \frac{y_1}{x_1} +\frac{y_2}{x_2}\\ =arg z_1 +arg z_2\\ \therefore arg (z_1z_2) =arg z_1 +arg z_2  \\

জটিল

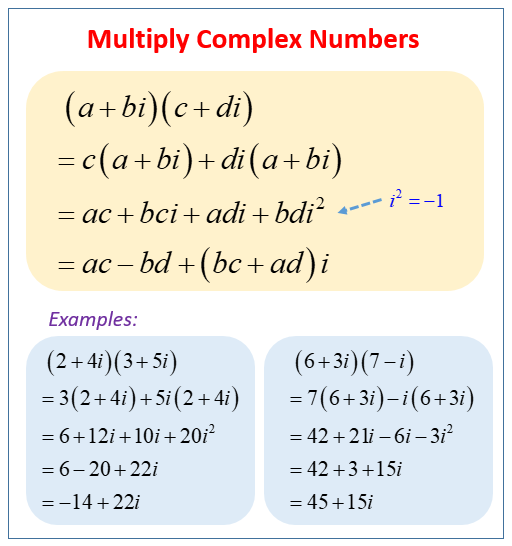
পোলার ব্যবস্থায় গুণ (Multiplication in Polar system)

ধরি, $z_1=r_1(\cos \theta_1 +i \sin \theta_1)$

এবং, $z_2=r_2(\cos \theta_2 +i \sin \theta_2)$

\therefore z_1z_2=r_1r_2(\cos \theta_1 \cos \theta_2 +i\sin \theta_2 \cos \theta_1 +i\sin \theta_1 \cos \theta_2 -\sin \theta_1 \sin \theta_2)\\ =r_1r_2\{(\cos \theta_1 \cos \theta_2 -\sin \theta_1 \sin \theta_2) -i(\sin \theta_2 \cos \theta_1 +\sin \theta_1 \cos \theta_2)\}\\ =r_1r_2\{\cos (\theta_1+\theta_2)-i\sin (\theta_1+\theta_2)\}\\ \therefore |z_1z_2|=r_1r_2=|z_1|.|z_2|\\ \therefore arg (z_1z_2) =\theta_1+\theta_2=arg z_1 +arg z_2

ওয়েলার সমীকরণ দ্বারা:

ধরি, $z_1=r_1e^{i\theta_1}$

এবং, $z_2=r_2e^{i\theta_2}$

\therefore z_1z_2=r_1r_2.e^{i\theta_1}.e^{i\theta_2}\\ =r_1 r_2.e^{i(\theta_1+\theta_2)}\\ \therefore |z_1z_2|=r_1r_2=|z_1|.|z_2|\\ \therefore arg (z_1z_2) =\theta_1+\theta_2=arg z_1 + arg z_2

∴ দুইটি জটিল সংখ্যার গুণফলের মডুলাস সংখ্যাদ্বয়ের মডুলাসের গুণফলের সমান এবং তাদের গুণফলের আর্গুমেণ্ট তাদের আর্গুমেন্টের সমষ্টির সমান।

জটিল সংখ্যার ভাগ (Division of complex numbers):

ধরি, $z_{1}=x_{1}+i y_{1}$

এবং, $z_{2}=x_{2}+i y_{2}$

\therefore \frac{z_{1}}{z_{2}}=\frac{x_{1}+i y_{1}}{x_{2}+i y_{2}}=\frac{\left(x_{1}+i y_{1}\right)\left(x_{2}-i y_{2}\right)}{\left(x_{2}+i y_{2}\right)\left(x_{2}-i y_{2}\right)}\\ =\frac{x_{1} x_{2}-x_{1} y_{2} i+x_{2} y_{1} i+y_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}\\ =\frac{x_{1} x_{2}+y_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}+i \frac{x_{2} y_{1}-x_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}\\ \therefore\left|\frac{z_{1}}{z_{2}}\right|=\sqrt{\left(\frac{x_{1} x_{2}+y_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}\right)^{2}+\left(\frac{x_{2} y_{1}-x_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}\right)^{2}}\\ \Rightarrow\left|\frac{z_{1}}{z_{2}}\right|^{2}=\frac{x_{1}{ }^{2} x_{2}{ }^{2}+y_{1}{ }^{2} y_{2}{ }^{2}+2 x_{1} y_{1} x_{2} y_{2}+x_{1}{ }^{2} y_{2}{ }^{2}+y_{1}{ }^{2} x_{2}{ }^{2}-2 x_{1} x_{2} y_{1} y_{2}}{\left(x_{2}{ }^{2}+y_{2}{ }^{2}\right)^{2}}\\ =\frac{x_{1}^{2} x_{2}^{2}+y_{1}^{2} y_{2}^{2}+x_{1}^{2} y_{2}^{2}+y_{1}^{2} x_{2}^{2}}{\left(x_{2}^{2}+y_{2}^{2}\right)^{2}}\\ =\frac{x_{1}{ }^{2}\left(x_{2}{ }^{2}+y_{2}^{2}\right)+y_{1}{ }^{2}\left(x_{2}{ }^{2}+y_{2}{ }^{2}\right)}{\left(x_{2}{ }^{2}+y_{2}^{2}\right)^{2}}\\ =\frac{\left(x_{1}{ }^{2}+y_{1}^{2}\right)\left(x_{2}^{2}+y_{2}^{2}\right)}{\left(x_{2}^{2}+y_{2}^{2}\right)^{2}}\\ =\frac{x_{1}^{2}+y_{1}^{2}}{x_{2}^{2}+y_{2}^{2}}\\ \therefore\left|\frac{z_{1}}{z_{2}}\right|=\frac{\sqrt{x_{1}^{2}+y_{1}^{2}}}{\sqrt{x_{2}^{2}+y_{2}^{2}}}\\ \therefore\left|\frac{z_{1}}{z_{2}}\right|=\frac{\left|z_{1}\right|}{\left|z_{2}\right|}\\ \therefore \arg \left(\frac{z_{1}}{z_{2}}\right)=\tan ^{-1} \frac{\frac{x_{2} y_{1}-x_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}}{\frac{x_{1} x_{2}+y_{1} y_{2}}{x_{2}^{2}+y_{2}^{2}}}=\tan ^{-1} \frac{x_{2} y_{1}-x_{1} y_{2}}{x_{1} x_{2}+y_{1} y_{2}}\\ =\tan ^{-1} \frac{\frac{x_{1} y_{2}-y_{1} x_{2}}{x_{1} x_{2}}}{\frac{x_{1} x_{2}+y_{1} y_{2}}{x_{1} x_{2}}}\\ =\tan ^{-1} \frac{\frac{y_{2}}{x_{2}}-\frac{y_{1}}{x_{1}}}{1+\frac{y_{1}}{x_{1}} \cdot \frac{y_{2}}{x_{2}}}\\ =\tan ^{-1} \frac{y_{1}}{x_{1}}-\tan ^{-1} \frac{y_{2}}{x_{2}}\\ =\arg z_{1}-\arg z_{2}\\

পোলার ব্যবস্থায় ভাগ (Division in Polar system):

ধরি, $z_{1}=r_{1}\left(\cos \theta_{1}+i \sin \theta_{1}\right)$

এবং, $z_{2}=r_{2}\left(\cos \theta_{2}+i \sin \theta_{2}\right)$

\therefore \frac{z_{1}}{z_{2}}=\frac{r_{1}}{r_{2}} \times \frac{\cos \theta_{1}+i \sin \theta_{1}}{\cos \theta_{2}+i \sin \theta_{2}}\\ =\frac{r_{1}}{r_{2}} \times \frac{\left(\cos \theta_{1}+i \sin \theta_{1}\right)\left(\cos \theta_{2}-i \sin \theta_{2}\right)}{\left(\cos \theta_{2}+i \sin \theta_{2}\right)\left(\cos \theta_{2}-i \sin \theta_{2}\right)}\\ =\frac{r_{1}}{r_{2}} \times \frac{\cos \theta_{1} \cos \theta_{2}-i \sin \theta_{2} \cos \theta_{1}+i \sin \theta_{1} \cos \theta_{2}+\sin \theta_{1} \sin \theta_{2}}{\left(\cos ^{2} \theta_{2}-i^{2} \sin ^{2} \theta_{2}\right)}\\ =\frac{r_{1}}{r_{2}} \times \frac{\cos \theta_{1} \cos \theta_{2}-i \cos \theta_{1} \sin \theta_{2}+i \sin \theta_{1} \cos \theta_{2}+\sin \theta_{1} \sin \theta_{2}}{1}\\ =\frac{r_{1}}{r_{2}} \times\left\{\left(\cos \theta_{1} \cos \theta_{2}+\sin \theta_{1} \sin \theta_{2}\right)+i\left(\sin \theta_{1} \cos \theta_{2}-\sin \theta_{2} \cos \theta_{1}\right)\right\}\\ =\frac{r_{1}}{r_{2}} \times\left\{\cos \left(\theta_{1}-\theta_{2}\right)+i \sin \left(\theta_{1}-\theta_{2}\right)\right\}\\ \therefore \frac{z_{1}}{z_{2}}=\frac{r_{1}}{r_{2}} \quad \therefore \arg \left(\frac{z_{1}}{z_{2}}\right)=\theta_{1}-\theta_{2}\\ \Rightarrow\left|\frac{z_{1}}{z_{2}}\right|=\frac{\left|z_{1}\right|}{\left|z_{2}\right|} \quad \therefore \arg \left(\frac{z_{1}}{z_{2}}\right)=\arg z_{1}-\arg z_{2}

ওয়েলার সমীকরণ দ্বারা:

ধরি, $z_{1}=r_{1} e^{i \theta_{1}}$

এবং, $z_{2}=r_{2} e^{i \theta_{2}}$

\therefore \frac{z_{1}}{z_{2}}=\frac{r_{1}}{r_{2}} \times \frac{e^{i \theta_{1}}}{e^{i \theta_{2}}}=\frac{r_{1}}{r_{2}} \times e^{i\left(\theta_{1}-\theta_{2}\right)}\\ \Rightarrow\left|\frac{z_{1}}{z_{2}}\right|=\frac{\left|z_{1}\right|}{\left|z_{2}\right|} \quad \therefore \arg \left(\frac{z_{1}}{z_{2}}\right)=\arg z_{1}-\arg z_{2}

∴ দুইটি জটিল সংখ্যার ভাগফলের মডুলাস সংখ্যাদ্বয়ের মডুলাসের ভাগফলের সমান এবং তাদের ভাগফলের আর্গুমেণ্ট তাদের আর্গুমেন্টের বিয়োগফলের সমান।

এইচএসসি ও এডমিশন পরীক্ষার্থীদের জন্য আমাদের কোর্সসমূহঃ

আমাদের স্কিল ডেভেলপমেন্ট কোর্সসমূহঃ

১০ মিনিট স্কুলের ক্লাসগুলো অনুসরণ করতে ভিজিট: www.10minuteschool.com

Other Topics