লিমিট (Limit)

লিমিট কি? (Limit)

$y=f(x)$ এর $x$ চলকেরে মান একটি নির্দিষ্ট ধ্রুব সংখ্যা $a$ অপেক্ষা বৃহত্তর বা ক্ষুদ্রতর মানগুলি গ্রহণ করে ক্রমশ $a$ এর নিকটবর্তী হওয়ায় যদি $f (x)$ ফাংশনের মান আরেকটি ধ্রুবক l এর নিকটবর্তী হয় তবে $1$ কে $f (x)$ ফাংশনের সীমা/limit বলে।

$\Rightarrow$ একে প্রকাশ করা হয় $l t / \lim$ ⁡দ্বারা।

$\operatorname{Lim} f(x)=l \rightarrow$ সীমাস্থমান।

যেমনঃ

$f(x)$ = $\frac{x^{2}-4}{x-2}$

$\frac{\lim }{x \rightarrow 0} f(x)$ = $\frac{\lim }{x \rightarrow 0} \frac{x^{2}-4}{x-2}$ ⁡

$x$	$f(x)$
2	$\alpha$
1.9	3.9
1.9999	3.9999
2.01	4.01
2.000001	4.000001

লিমিট

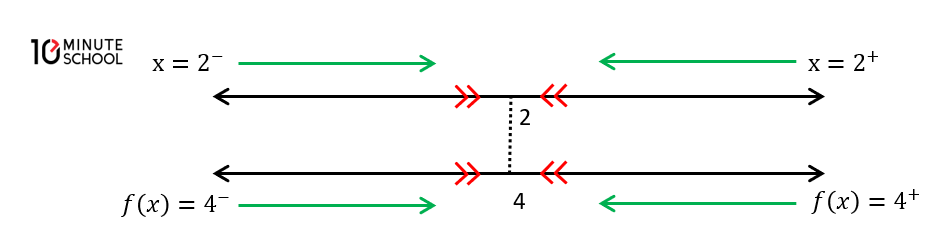
⟹ বামদিকবর্তী লিমিট (The limit on the left) :

$x$ চলক $a$ অপেক্ষায় ক্ষুদ্রতম মান গ্রহন করে বামদিক হতে ক্রমশ $a$ এর নিকটবর্তী হওয়ায় যদি $f (x)$ ফাংশনের মান ধ্রুবক $1$ এর নিকটবর্তী হয় তবে $1$ কে $f (x)$ ফাংশনের বামদিকবর্তী বা বামাবর্তী লিমিট বলে।

$\frac{\operatorname{Lim}}{x \rightarrow a^{-}} f(x)$ = $l$

⟹ ডানদিকবর্তী লিমিট (The limit on the right) :

$x$ চলক $a$ অপেক্ষায় বৃহত্তম মানগুলি গ্রহন করে ডানদিক হতে ক্রমশ $a$ ‘ এর নিকটবর্তী হওয়ায় যদি $f (x)$ ফাংশনের মান ধ্রুবক $1$ এর নিকটবর্তী হয় তবে $1$ কে $f (x)$ ফাংশনের ডানদিকবর্তী বা ডানাবর্তী লিমিট বলে।

$\frac{\operatorname{Lim}}{x \rightarrow a^{+}} f(x)$ = $l$

⟹ সীমার মৌলিক ধর্মাবলী (The basic religions of the range):

$\frac{\operatorname{lim}}{x \rightarrow a} f(x)=$ $l$ এবং $\frac{\operatorname{Lim}}{x \rightarrow a} g(x)$ = $m$

1. $\frac{\operatorname{lim}}{x \rightarrow a}\{f(x) \pm g(x)\}$ = $\frac{\operatorname{Lim}}{x \rightarrow a} f(x) \pm \frac{\operatorname{Lim}}{x \rightarrow a} g(x) j$ = $l \pm m$

2. $\frac{\operatorname{lim}}{x \rightarrow a} c f(x)$ = $c \frac{\operatorname{Lim}}{x \rightarrow a} f(x)$ ; c=ধ্রুবক

3. $\frac{\operatorname{lim}}{x \rightarrow a} f(x) g(x)$ = $\frac{\operatorname{Lim}}{x \rightarrow a} f(x) \cdot \frac{\operatorname{Lim}}{x \rightarrow a} g(x)$ = $\operatorname{lm}$

4. $\frac{\lim }{x \rightarrow a} \frac{1}{f(x)}$ = $\frac{1}{l}$ ; if $l \neq 0$

5. $\frac{\operatorname{lim}}{x \rightarrow a} \frac{f(x)}{g(x)}=\frac{l}{m} ;$ if $m \neq 0$

6. $\frac{\operatorname{lim}}{x \rightarrow a} c=c ; \mathrm{c}= \text {ধ্রুবক}$

এইচএসসি ও এডমিশন পরীক্ষার্থীদের জন্য আমাদের কোর্সসমূহঃ

আমাদের স্কিল ডেভেলপমেন্ট কোর্সসমূহঃ

১০ মিনিট স্কুলের ক্লাসগুলো অনুসরণ করতে ভিজিট: www.10minuteschool.com

Other Topics